The new energy dissipation model implemented in RAMMS::ROCKFALL (not yet released) are based on the work energy theroem for rigid bodies impacting compactable soft soils. For the full story please consult this publication. The most important features are shown below.
The Work-Energy Theroem ¶
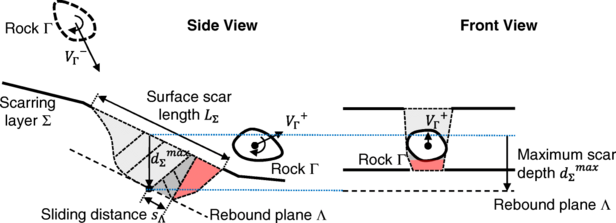
To simulate rock-ground interaction, the terrain is divided into a plastic, deformable scarring layer Σ and a non-deformable, hard contact ‘slippage’ plane Λ. Both the scarring layer Σ and the rebound plane Λ are located below the digital elevation model. This division is the core assumption of the model.
To find the appropriate form of the drag resistance, we apply the work energy theorem to the collisional system consisting of the rock Γ and the scarring layer Σ, see Fig. 2. Consider first the case of a rock’s vertical drop onto a horizontal ground forming an entirely plastic collision, where the rock does not rebound out of the soil substrate and all the kinetic energy is dissipated. To simplify our derivation, we assume that the work that gravity does to the rock along the braking distance dΣ is much smaller than that of the braking force FId and thus can be omitted. In this case, the change in rock kinetic energy ΔKΓ is proportional to the product of FId and dΣ:
$$ \Delta K_{\Lambda} = \frac{1}{2}M_{\Lambda}V_{\Lambda}^2 – 0 = \left(F_d^I – F_g^I\right) d_{\Sigma} \approx F_d^I d_{\Sigma} = \int_0^t F_d^I(t) ||\dot{\zeta_{\Gamma}}(t) || dt $$
where $ V_{\Gamma} $ corresponds to the speed of rock right before it contacts with the ground surface. Here, we also assume that $ F_d^I $ acts along the vertical direction; thus, $ d_{\Sigma} $ is equivalent to the maximum rock penetration depth $ d_{\Sigma}^{\mathrm{max}} $. We now express the drag force in the soil substrate as a velocity squared resistance parameterised by the scarring coefficient $ C_d^I $, which is equivalent in form to drag defined in fluid dynamics:
$$ F_d^I(t) = \frac{1}{2}C_d^I\rho_{\Sigma}A_{\Gamma}(t)||\dot{\zeta_{\Gamma}}(t)||^2 $$
where $ A_{\Gamma}(t) $ is the time dependent cross-sectional area of the penetration scar. The significant conclusion from this analysis is that (1) the drag force acting on the rock will be parameterised as a velocity squared dependent drag, (2) the drag coefficient physically represents the ratio between the characteristic volumes of the rock and the scar and (3) the soil compacting rate can influence the drag coefficient. At least $ C_d^I $ can be approximated from field observations where maximum penetration depth $ d_{\Sigma}^{\mathrm{max}} $ and scar length $ l_{\Sigma} $ can be measured and compared with the characteristic length of the rock $ d_{\Gamma} $
Scarring Phase ¶
The rock $ \Gamma $ enters the scarring layer $ \Sigma $. The rock is penetrating a vertical distance $ \zeta\Gamma(t) $ until it reaches the rebound plane. The velocity of the rock at this stage is $ V_{\Gamma \rightarrow \Sigma}$. The sliding phase begins when the penetration depth is equal to the maximum scar depth $ \zeta\Gamma(t) = d_{\Sigma}^{\mathrm{max}} $. The scarring drag force experienced by the rock in the scarring layer is proportional to its frontal effective area $ A_{\Gamma}(t) $, i.e. the projected area of the rock-soil intersecting body onto a plane that is perpendicular to the rock velocity. Note that $ A_{\Gamma(t)} $ depends on rock orientation, velocity direction and penetration depth.

The above figure illustrates the process that a rock Γ enters the scarring layer $ \Sigma $. Right before the rock $ \Gamma $ enters the scarring layer $ \Sigma $, the maximum scar depth (rebound depth) dmax $ \Sigma $ that can be generated by this impact is evaluated. Importantly, $ d_{\Sigma}^{\mathrm{max}} $ relies not only on the mechanical strength $ M_{\Sigma}^{e} $ of the ground material but also on the mass, collisional speed, and even the orientation of the nonspherical rock at impact. To this end, Gerber (2019) proposed a rock penetration equation obtained through fitting the experimental data for freely, vertically dropped rocks of various masses and initial heights, and considering Hertz theory for the contact forces calculation. This formula reads as follows:
$$ d_{max} = 0.1 \cdot M_{\Gamma}^{\frac{1}{3}} \cdot M_{e_{\Sigma}}^{0.4} \cdot ||V_{\Gamma}^{\perp}||^{0.8} $$
where $ d_{\Sigma}^{\mathrm{max}} $ is the maximum rock penetration depth (rebound depth), $ M_{\Gamma} $ is the mass of rock, $ M_{\Sigma}^{e} $ is the mechanical strength of soil and $ V_{\Gamma}^{\perp} $ is the vertical component of rock velocity corresponds to the speed right before it touches ground surface. This equation determines consequently the maximal penetration depth. Note that the parameter $ M_{\Sigma}^{e} $ has a unit kN/m2.
When the rock comes into contact on the top of terrain surface, the soil layer, suffering from the external pressure, ‘flows’ around the rock-ground intersecting faces. This flow of the surrounding fine particles, alike fluid, exerts a drag force against the rock motion ( Blasio et al. 2018). On the other hand, upon rock collision, the ground can be compacted. This compaction process normally leads to an increase in drag force, i.e. the drag coefficient grows as a result of particle jamming ( Albert et al. 2001). We therefore divide the scarring drag coefficient into two processes shown by:
$$ C_d^I = C_f^I + C_s^I $$
where $ C_f^I $ is the drag coefficient accounting for the fluid behaviour of soil, and $C_s^I $ is the additional drag coefficient taking into account of the soil compaction. $C_s^I $ varies with soil mechanical strength $ M_{\Sigma}^{e} $ and rock mass $ M_{\Gamma} $ . On one hand, for a given $ M_{\Sigma}^{e} $ value, we anticipate a higher $C_s^I $ for a larger $ M_{\Gamma} $ , which in general corresponds to a deeper penetration depth $ d_{\Sigma}^{\mathrm{max}} $. On the other hand, for a given $ M_{\Gamma} $ value, one expects that $C_s^I $ decreases with decreasing $ M_{\Sigma}^{e} $ as it is more difficult to build up a high resultant stress and thus a strong local plastic yielding in a looser granular media.
Sliding Phase ¶
Sliding phase: the rock $ \Gamma $ slides along the rebound plane $ \Lambda $. This process begins when the penetration depth is equal to the maximum scar depth $ \zeta_{\Gamma}(t) = d_{\Sigma}^{\mathrm{max}} $ . The frictional coefficient increases while rock slips on the rebound plane $ \Lambda $. The velocity of the rock at this stage is $ V_{\Gamma \rightarrow \Lambda} $. The sliding phase ends when the contact of rock with the rebound plane is broken.
$$ \mu_{\Lambda}(s_{\Lambda}) = \mu_{\Lambda}^{min} + \frac{2}{\pi} \cdot \left(\mu_{\Lambda}^{max} – \mu_{\Lambda}^{min} \right) \cdot \arctan(\kappa_{\Lambda}s_{\Lambda}) $$

Rebounding Phase ¶
Rebounding phase: the rock $ \Gamma $. rebounds. Scarring drag still acts on the rock during the rebounding phase, which ends when the rock completely detaches from the scarring layer $ \Sigma $.